IGCSE Mathematics: Mastering Quadratic Equations

Quadratic equations are a fundamental topic in IGCSE Mathematics. Understanding how to solve and apply them is essential for success in various areas of math and real-world problem-solving. In this article, we’ll explore the basic form of quadratic equations, methods of solving them, and how they can be applied to everyday problems.

Quadratic Equations

What is a Quadratic Equation?

A quadratic equation is a polynomial equation of the form:ax2+bx+c=0ax^2 + bx + c = 0ax2+bx+c=0

where aaa, bbb, and ccc are constants, and xxx represents an unknown variable. The highest degree (or exponent) of xxx is 2, which defines it as a quadratic equation.

• If a=0a = 0a=0, the equation becomes linear.
• If a≠0a \neq 0a=0, the equation forms a parabola when graphed.

• Introduction to Quadratic Equations – BBC Bitesize

Solving Quadratic Equations

There are several methods to solve quadratic equations, each with its advantages depending on the specific equation.

1. Factoring: Factoring is one of the simplest methods, but it only works for certain equations. You rewrite the quadratic equation in a factorized form:ax2+bx+c=(mx+n)(px+q)=0ax^2 + bx + c = (mx + n)(px + q) = 0ax2+bx+c=(mx+n)(px+q)=0To solve the equation, set each factor to zero and solve for xxx:(mx+n)=0or(px+q)=0(mx + n) = 0 \quad \text{or} \quad (px + q) = 0(mx+n)=0or(px+q)=0Example:x2−5x+6=0⇒(x−2)(x−3)=0x^2 – 5x + 6 = 0 \quad \Rightarrow \quad (x – 2)(x – 3) = 0x2−5x+6=0⇒(x−2)(x−3)=0The solutions are x=2x = 2x=2 and x=3x = 3x=3.

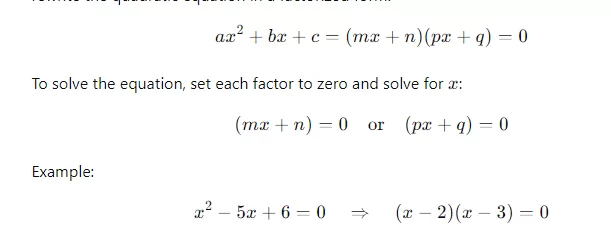
1. Factoring Quadratics – Khan Academy
2. Completing the Square: Completing the square is a method that transforms the quadratic equation into a perfect square trinomial:ax2+bx+c=(x+p)2=qax^2 + bx + c = (x + p)^2 = qax2+bx+c=(x+p)2=qThis method is particularly useful for equations that cannot be easily factored. Once in this form, solve for xxx by taking the square root of both sides.Example:x2+6x+9=0⇒(x+3)2=0x^2 + 6x + 9 = 0 \quad \Rightarrow \quad (x + 3)^2 = 0x2+6x+9=0⇒(x+3)2=0The solution is x=−3x = -3x=−3.

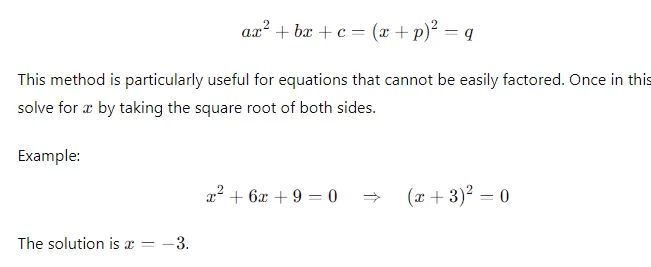
Completing the Square – Math Is Fun

1. Quadratic Formula: The quadratic formula is a universal method that works for any quadratic equation:x=−b±b2−4ac2ax = \frac{-b \pm \sqrt{b^2 – 4ac}}{2a}x=2a−b±b2−4ac​​Here, the discriminant (b2−4acb^2 – 4acb2−4ac) determines the nature of the solutions:
   • If b2−4ac>0b^2 – 4ac > 0b2−4ac>0, there are two real solutions.If b2−4ac=0b^2 – 4ac = 0b2−4ac=0, there is one real solution.If b2−4ac<0b^2 – 4ac < 0b2−4ac<0, there are two complex (imaginary) solutions.
   Example:2×2+3x−2=0⇒x=−3±32−4(2)(−2)2(2)2x^2 + 3x – 2 = 0 \quad \Rightarrow \quad x = \frac{-3 \pm \sqrt{3^2 – 4(2)(-2)}}{2(2)}2×2+3x−2=0⇒x=2(2)−3±32−4(2)(−2)​​The solutions are x=−3+254=0.5x = \frac{-3 + \sqrt{25}}{4} = 0.5x=4−3+25​​=0.5 and x=−3−254=−2x = \frac{-3 – \sqrt{25}}{4} = -2x=4−3−25​​=−2.

1. Quadratic Formula – BBC Bitesize

The Discriminant

The discriminant (b2−4acb^2 – 4acb2−4ac) is an important part of the quadratic formula because it tells you how many solutions the equation has, and whether those solutions are real or complex.

1. Understanding the Discriminant – Varsity Tutors

Graphing Quadratic Equations

Quadratic equations are often represented graphically as parabolas. The general shape of a parabola depends on the value of aaa:

• If a>0a > 0a>0, the parabola opens upwards (U-shaped).
• If a<0a < 0a<0, the parabola opens downwards (inverted U-shaped).

Key features of a parabola include:

• Vertex: The highest or lowest point on the graph.
• Axis of Symmetry: The vertical line that passes through the vertex.
• Roots or x-intercepts: The points where the parabola intersects the x-axis (i.e., the solutions of the equation).

The vertex of a parabola can be found using the formula:xvertex=−b2ax_{\text{vertex}} = \frac{-b}{2a}xvertex​=2a−b​

• Graphing Quadratics – Math Planet

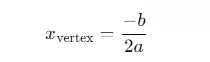
Applications of Quadratic Equations

Quadratic equations are used in a variety of real-world scenarios:

1. Projectile Motion: The path of a projectile (like a ball being thrown) follows a quadratic equation, where the height of the object is a function of time. Example: The equation h(t)=−4.9t2+vt+h0h(t) = -4.9t^2 + vt + h_0h(t)=−4.9t2+vt+h0​ describes the height of a projectile in terms of time ttt, initial velocity vvv, and initial height h0h_0h0​.
2. Optimization Problems: Quadratic equations are often used to find maximum or minimum values in optimization problems, such as finding the best price to sell a product or determining the maximum area for a given perimeter.
3. Area Calculations: Quadratics can be used to calculate areas of geometric shapes when they involve squared dimensions.
   • Quadratic Equations in Real Life – ThoughtCo

Conclusion

Quadratic equations are a critical component of IGCSE Mathematics. Mastering the different methods of solving quadratics, understanding the discriminant, and learning how to apply them in real-life situations will help you succeed in math and beyond. Whether through factoring, completing the square, or using the quadratic formula, you’ll have the tools you need to solve any quadratic equation with confidence.